Statistical physics and path integral quantization rely on a precise counting of the different states and configurations of a system. In particular, quantum gravity involves studying the possible topology and geometry of space-time. This implies deep relations between physics and topology, combinatorics and geometry, as well as with probability theory. Some seminal contributions originated from IPhT, and the Institute has a number of experts in the field, whose activity covers a large spectrum of approaches.
Dimers, clustered algebras and beyond
A symmetric tiling problem
Dimer models
Dimer models are archetypical examples of statistical models with strong underlying geometric constraints. Those models have natural reformulations as tilings of two-dimensional domains. On nice domains, with suitable boundary conditions, such tilings exhibit an arctic curve phenomenon where the system displays a sharp separation between frozen phases near the boundary and fluid phases away from the boundary, not unlike a freezing pond. Several methods were used at IPhT to derive arctic curves: (1) Using exact solutions of cluster algebra mutation relations for the T-system, which are generally expressed as dimer partition functions over domains determined by the initial conditions, in order to calculate the local dimer density, whose singularities determine the arctic curves; (2) Applying more generally the “tangent method” which gives access to the arctic curve by computing boundary one-point functions. The latter was successfully applied to interacting fermion models as well, such as integrable 6 and 20 Vertex models with special “domain wall” boundary conditions. Remarkable relations were also established by IPhT researchers between different enumeration problems, such as that between the 20 Vertex model and the domino tiling of some suitably symmetrized domain.
Cluster algebras
Cluster algebras, invented as a pure combinatorial structure, found many applications in mathematics (representation theory, geometry…) and statistical physics. In a nutshell, a cluster algebra is a multi-time dynamical system describing the evolution of variables via mutations. Among other contributions, a team at IPhT explored the link between the cluster algebra structure of the solutions to the abstract T-system satisfied by transfer matrices of integrable quantum spin chains and the statistical mechanics of dimer models on certain graphs called networks. In particular, partition functions on fixed domains correspond to mutation invariants. A quantized cluster algebra structure was used to compute graded tensor product multiplicities of representations occurring in inhomogeneous quantum spin chains, generalizing the difference Toda equation. The same structure allows to rephrase the theory of Macdonald difference operators (of which Macdonald polynomials are common eigenfunctions), in relation to symmetries of the underlying Double Affine Hecke Algebras, for all classical types, as well as for genus two.
Another important IPhT activity standing at the intersection of statistical mechanics, discrete geometry and integrable systems consists in developing theories that encompass both exactly solvable models of 2D statistical mechanics (dimer model, Ising model, etc) and discrete integrable dynamics arising in geometry (pentagram map, Q-net propagation, etc). This activity also uses tools from combinatorics (cluster algebras, graph theory, etc).
Random maps
Maps are discrete surfaces made of polygons glued side by side. Random maps are used as a simplified model of quantum gravity, and their continuous limit is a model of random surfaces in statistical physics. The problem of enumerating random discrete surfaces has been solved in the easiest cases, but has remained a challenge for surfaces carrying matter (Ising spins) or colors, or with higher topology of non-trivial boundary conditions, or with fixed geodesic lengths of some paths, and in general with more structure.
The bijective approach to card enumeration
A remarkably efficient map enumeration technique method consists in establishing a one-to-one correspondence between planar maps and some kinds of genealogical trees, called mobiles. Originally limited to quadrangulations, this correspondence was then extended to all maps via the so-called BDG (Bouttier Di Francesco Guitter) bijection. This coding simplifies drastically the enumeration of maps and the bijective approach eventually allowed one to access very refined enumeration results on the random geometry, such as the profile of distances which gives the distribution law for the distance between two random vertices chosen uniformly on the (itself random) map. Another related bijective coding of random maps is the “slice decomposition” which consists in cutting the maps into elementary blocks, the so-called slices. Recently, the slice decomposition of planar maps with three boundaries by IPhT researchers revealed the deep analogy between the enumeration of maps and hyperbolic geometry.
Maps and integrable systems
In 2023, a team at IPhT proved a longstanding conjecture and long expected by the community of combinatorists, giving the number of mobiles (that are trees which count planar maps with geodesic lengths). The formula coincides with the Tau function of a highly degenerate integrable system, and the proof involves a deep interplay between integrable systems methods and combinatorial methods. In 2021, IPhT researchers solved a combinatorial model, that was introduced conjecturally by M. Kontsevich for the r-spin Witten conjecture. R-spin intersection numbers play an important role in algebraic geometry and topological string theory. The problem is to count (measure the volume of) the moduli space of Riemann surfaces, of given genus and marked points, equipped with a r-spin structure (a fractional differential form whose r-th power is a 1-form). Witten had conjectured that the generating function of these numbers is an r-KdV Tau-function. The case r=2, was proved by M. Kontsevich, who decomposed the moduli spaces in cells labeled by graphs, and introduced a model of random maps for intersection numbers. For the r-spin case, M. Kontsevich also conjectured a graph model. A team of the Institute studied the combinatorics of these r-spin graphs, and by recursion on the number of edges, was able to compute explicitly the generating functions, showing that they obey the topological recursion, and showing that they obey the r-KdV equations. Besides this is a beautiful model of random maps.
Liouville quantum gravity, SLE and CLE
An area of research being intensively studied at the IPhT in collaboration with mathematicians from Cambridge (UK and USA) deals with the conformal random geometry of maps decorated by statistical mechanics models. Their common continuous limit must be described by random surfaces in Liouville quantum gravity (LQG), both as a random measure and metric space, and by the random paths of Schramm-Loewner evolutions (SLE) and conformal loop sets (CLE). The natural coupling between SLE and LQG and the use of the rigorous Knizhnik-Polyakov-Zamolodchikov (KPZ) relation give a conformally invariant description of this continuous limit. In particular, the ‘tree-coupling’ approach to Liouville quantum gravity provided the first rigorous proof that random maps decorated by Fortuin-Kasteleyn clusters converge to LQG surfaces decorated by a CLE, in the so-called ‘peanosphere’ topology. This approach also justifies the use of LQG and the KPZ relation to predict the exact shapes of all mean-integral multifractal spectra associated with the various versions of the SLE in the Euclidean plane, as well as the large deviation functions of the CLE.
Loop and meander models
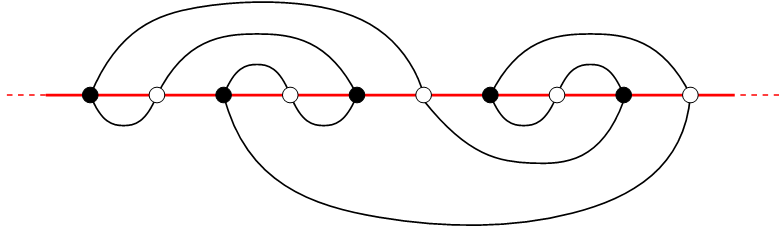
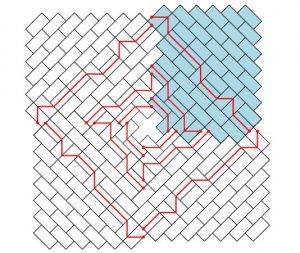
A meander configuration
The meander problem is a particular example of a model of compact loops on random maps, i.e. statistical systems of maps equipped with a set of loops that avoid each other and visit all the vertices of the map. This last condition induces strong geometric correlations between the statistical set of loops and the set of underlying random maps, which makes the question of the universality of these systems much more subtle than for the usual loop models. A particular direction of the study carried out at the IPhT is to establish a classification of possible universality classes for compact loop models and to understand the relevant criteria that make the system fall into one or other universality class. Particular attention has also been paid to characterising loop systems by predicting their configuration exponents and contact exponents.
Topological recursion
topological recursion is a universal recurrence which, starting from a “spectral curve” (an algebraic curve with an additional structure), produces a double sequence of differential forms Wg,n. This recursion is satisfied by many enumerative surface counting problems, as well as by many correlation functions in random matrix theory, integrable systems and string theory. Some questions about topological recursion concern applications of this recursion: proving that a certain model (from integrable systems, random matrices, card combinatorics, string theory, etc.) satisfies topological recursion, and then using it to compute correlation functions. Another question concerns the theory of topological recursion itself: the aim is to prove certain universal properties. One major conjecture is to prove that topological recursion provides the WKB expansion of wave functions of integrable systems: this conjecture is often called the “quantum curve conjecture”. It had already been proved in the simplest cases (rational spectral curves) and a team at the IPhT proved it for elliptic curves, then hyperelliptic curves, then almost general algebraic curves.
Random Delaunay triangulations

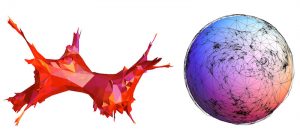
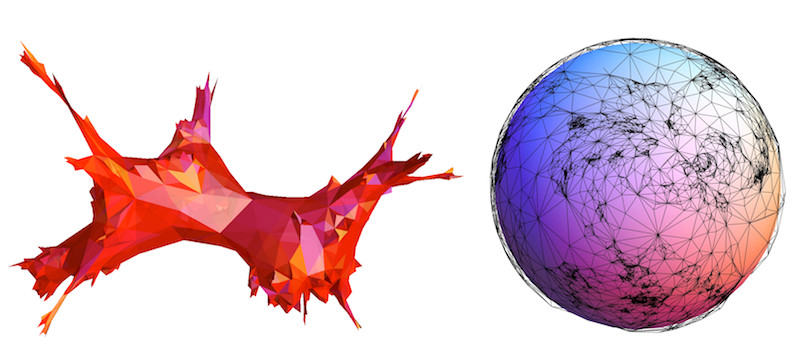
Samples of “uniform” (left) and “2d gravity-like” (right) random Delaunay triangulations
The Random Planar Delaunay triangulation model was proposed by an IPhT team in 2013. It is an extension of random map models for two-dimensional quantum gravity, with explicit conformal invariance at the discrete level. It is related to circle pattern problems, and to many structures studied in related contexts (combinatorics, maps and integrable systems, cluster algebras, hyperbolic geometry, etc). Recent studies by IPhT (with Brandeis U.) focus on the relation of the model with deformations of isoradial triangulations, the emergence of a discrete stress energy tensor, and its relation to discrete analyticity in other discrete models of statistical mechanics.
Scientific staff
Emmanuel GUITTER
Olivier GOLINELLI
Jérémie BOUTTIER (on secondment)
Bertrand DUPLANTIER
Sanjay RAMASSAMY
Bertrand EYNARD
Philippe DI FRANCESCO
Dalimil MAZAC
Michel BAUER
Hubert SALEUR
Sylvain RIBAULT
Emeritus members & scientific advisers
André VOROS
François DAVID