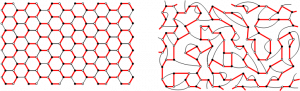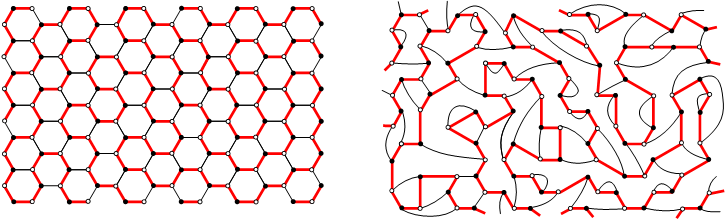
Examples of measured exponents and their comparison to KPZ predictions, before and after renormalization

    The famous KPZ formulas (Knizhnik-Polyakov-Zamolodchikov, 1988) relate the critical exponents of statistical models on regular two-dimensional lattices to the exponents of the same models on planar random lattices. These relations, verified exactly in a large number of statistical mechanics systems, have now acquired a rigorous mathematical status, under certain technical assumptions of statistical independence. Hamiltonian paths on lattices, which are self-avoiding paths forced to visit all the sites of the lattice, constitute a critical statistical model where the geometrical constraints are particularly strong. A team at IPhT studied the case of the honeycomb lattice and its random counterpart, the bicubic lattice (a bipartite lattice made only of trivalent vertices colored in black and white so that the vertices of one color are connected only to those of the other color). The critical exponents in the first case are computed by standard Coulomb gas methods, while the critical exponents in the second case can be obtained numerically with a high accuracy from the exact enumeration of Hamiltonian path configurations for finite size lattices.

Surprisingly, the expected KPZ relations fail for some types of critical exponents, indicating that a new mechanism is at work which goes beyond their usual scope of application! A procedure (heuristic at this stage) of renormalization (readjustment of the KPZ formulas with a new parameter) is proposed which seems to restore the validity of these relations. The challenge is now to understand mathematically how the particular geometrical constraints of the Hamiltonian paths can influence the statistics of the bipartite random lattice, and lead to such a renormalization.

Ref: P. Di Francesco, B. Duplantier, O. Golinelli and E. Guitter, Exponents for Hamiltonian paths on random bicubic maps and KPZ arXiv :2210/08887 [math-ph]