Introduction
Group C’s research activities focus on low-energy physics, from condensed matter to quantum information, including statistical physics and interdisciplinary applications. A common research focus is the study of strongly correlated, forced and/or disordered systems. At the classical level, these systems give rise to complex and rich behaviors, in particular the glassy and amorphous phases of matter. At the quantum level, phenomena range from high-temperature superconductivity to topological materials. Particular emphasis is placed on non-equilibrium systems, both classical and quantum, on the mechanisms underlying the absence or approach of thermalization, and on their description by stochastic processes, field theory techniques and advanced mean-field methods. Finally, applications of statistical physics to interdisciplinary issues such as learning problems, complex networks and biophysics are developed, as well as a research direction in quantum information, from the study of quantum computing architectures to communication protocols and quantum cryptography.
Non equilibrium and Disordered Systems
The new frontier in statistical physics is to build a general theory of systems out of equilibrium, continuously evolving with time. No theoretical framework is available that would encompass the physics of systems interacting with their environment through continuous exchanges of charge, spin, energy, or momentum. Yet, in nature, heat and matter fluxes are ubiquitous and systems far from equilibrium are the majority rather than the exception. Artificial devices (complex networks, urban patterns), algorithms currently used in data sciences, as well as living matter provide us with prominent examples. The IPhT is a major actor in the study of these crucial issues.
A global perspective of time-dependent processes involving a large number of interacting elementary constituents requires elaborate theoretical tools that can be developed using techniques of quantum field theory, conformal symmetries and integrable systems. Exact solutions of models far from equilibrium have allowed us to determine rare event statistics and large deviation functions that play the role of non-equilibrium thermodynamic potentials. We study phase transition in random systems such as structural glasses, as well as
their dynamics and relation to jammed packings, and to spin glasses.
All the above methods are applied very successfully to multidisciplinary subjects such as machine learning, statistics, analysis of neural-networks, theory of algorithms, and biology (e.g. structure and folding of bio-molecules). Finally, the quantitative study of geographic and urban networks using the mathematical apparatus of statistical mechanics is a rapidly developing theme that offers a promising cross-disciplinary perspective
Quantum Systems and Condensed Matter
At low temperatures, when quantum effects become important, condensed-matter systems can exhibit many spectacular phenomena. In this context, superconductivity, superfluidity, quantum Hall effect, quantum phase transitions, or the Anderson localization are a few classic examples. The understanding of these systems can be particularly challenging in presence of strong interactions among the particles (electrons, bosons, or spins), and/or in presence of disorder, or when not in thermal equilibrium.
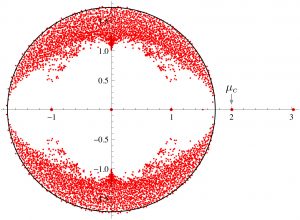
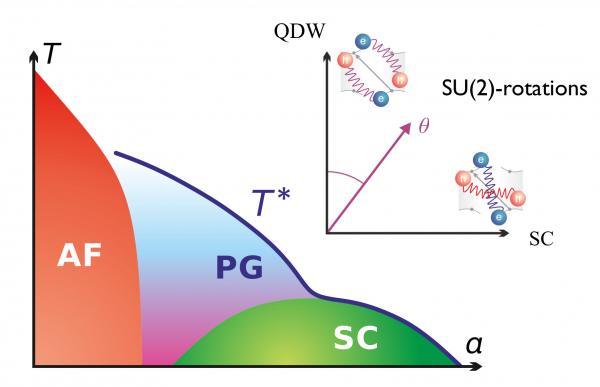
At the institute we use and develop a large spectrum of theoretical and/or numerical techniques to investigate these problems, like field theory, effective models, dynamical mean-field theory, integrability methods, or many-body simulations to name a few. In the last few years, the research activity at the IPhT has been focused on topological states of matter (Majorana Physics), high-temperature superconductivity, systems far from equilibrium (dissipation, periodic driving or quantum quenches), transport phenomena (impurity models and low-dimensional systems), as well as on the role of disorder (Many-body localization).
Soft Matter and Biological Systems
Polymers provide physical realizations of stochastic processes such as Brownian motion or self-avoiding random walks. Other types of stochastic processes control the functioning of molecular motors and the folding of proteins. Some universal aspects of membranes (flexible films, biological membranes) are closely related to the random geometries studied in string theories and quantum gravity. When objects are charged (polyelectrolytes, charged membranes) or possess internal degrees of freedom, their physical and geometrical properties may be deeply modified: new phases may appear. Random polymer physics governs the complex interactions between chemically different monomers in biopolymers. One can study the denaturation of DNA or protein folding and RNA within this framework. For the latter, the classification of folded forms can be done using tools of topology (genus, Euler characteristic), leading to the development of powerful algorithms for structure prediction. In addition, most biopolymers carry charges, and the Coulomb interaction determines their universal properties of aggregation and solvation in the cell.

Quantum information theory
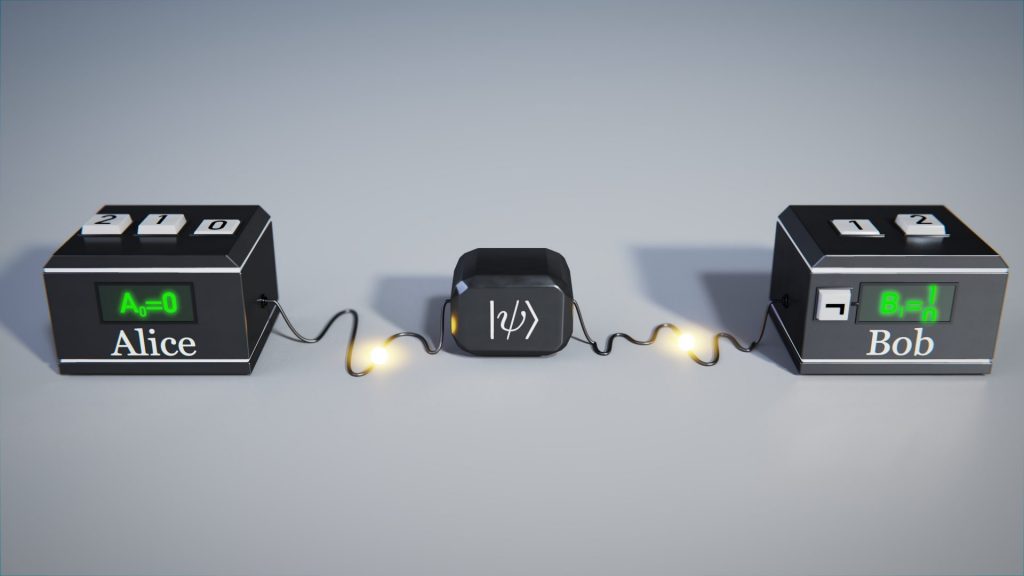
Information theory aims at studying and quantifying information to optimise its processing, storage and communication. Quantum information aims to understand what we gain in having information encoded in quantum systems that can be manipulated and measured individually. By offering processing units with no classical equivalence, quantum computers have emerged as one of the most promising applications of quantum information. While they offer unique opportunities for the efficient synthesis of chemical substances such as medicines or fertilisers for modern agriculture for example, quantum computers represent a threat for currently used classical crypto-systems. But quantum information also provides solutions for secure communication with an unprecedented security level, secure even against hackers having a quantum computer. All of these applications have emerged from interdisciplinary efforts involving quantum physicists, computer scientists working in complexity theory or mathematicians doing cryptography. Physicists working at IPhT quantify quantum resources that are needed for implementing future quantum technologies and develop theoretical tools to certify their proper functioning. They also have more fundamental activities aiming to study and quantify quantum correlations in large and complex systems and more broadly to explore fundamental questions to gain insights on quantum theory.