The first notable work of Michel Gaudin was an elegant demonstration of Wick's theorem in finite temperature, in 1960. This was followed by pioneering works in random matrix theory. With Madan Lal Mehta, he obtained the analytical expression for the density of eigenvalues of a random matrix, leading to the first derivation of the semicircle law. In this work, they introduced the orthogonal polynomials and the method of integration on alternating variables to deal with the case of orthogonal matrices. Next, he obtained the level-spacing law in excellent agreement with Wigner's hypothesis. This work introduced crucial tools in the field of random matrices that are still widely used, in particular the sine kernel and spheroidal functions to diagonalize the associated Fredholm equation. Its repercussions extended to number theory and the study of the zeros of the Riemann zeta function.
In the mid-sixties, inspired by the work of Edouard Brézin and Jean Zinn-Justin, he turned his attention to the problem of spin 1/2 fermions on a circle with a delta-function potential, extending the bosonic system considered by Lieb and Liniger. To solve this problem, he introduced the nested Bethe Ansatz, which proved to be a fundamental tool in the study of integrable systems. His PhD thesis, available in the collection of his works, "Modèles exactement résolus" (1995), explains his approach and contains between the lines the expression of the norm of Bethe's states, the solution of his famous model and no doubt other gems.
During his stay at the Stony Brook Institute for Theoretical Physics in 1970, at the invitation of C. N. Yang, he made important contributions to the study of a one-dimensional gas of bosons in delta-function potential. In particular, the study of the orthogonality properties of the scattering wave functions led him to introduce a determinant subsequently rediscovered and known as the Izergin and Korepin partition function. Using Coxeter groups, he also constructed a wave function generalizing Bethe's wave function to the case of open edges. In another essential work, inspired by the method of C. N. Yang and C. P. Yang for the thermodynamics of the boson system, he obtained, simultaneously with Takahashi, the thermodynamics of the Heisenberg chain.
A series of Gaudin’s works brought essential contributions to the field of solvable models. In 1976 he introduced and solved a family of quadratic Hamiltonians, now known as the "Gaudin Model". This model has become a classic in the theory of cold atoms and superconductivity. Its solution exploits a first, simple version of the Algebraic Bethe Ansatz. It has also been a source of inspiration for mathematicians working on the Langlands program.
In the 1970s, Gaudin faced also the problem of the exact solution of non-integrable systems. In particular, he and his student Bernard Derrida, proposed in 1975 a solution to the problem of three bodies in delta-function potential with different couplings.
His book "La fonction d'onde de Bethe" (Bethe's wave function), written in 1981, is still a reference in the field. It was translated into Russian in 1987 by P. Kulish and E. K. Sklyanin and translated in English in 2013 by Jean-Sébastien Caux.
Gaudin has also had several fruitful collaborations with other physicists at the IPhT. Let us mention in particular: the work with Ivan Kostov on the solution of O(n) model in quantum gravity; the work with Claude Itzykson, Philippe Di Francesco and Frédéric Lesage on the wave functions of the quantum Hall effect; the work with Denis Bernard, Duncan Haldane and Vincent Pasquier on the solution of the Haldane-Shastry chain; the work with Jacques Bros, Henri Epstein and Ugo Moschella on the geometrically-induced instability of particles in de Sitter space.
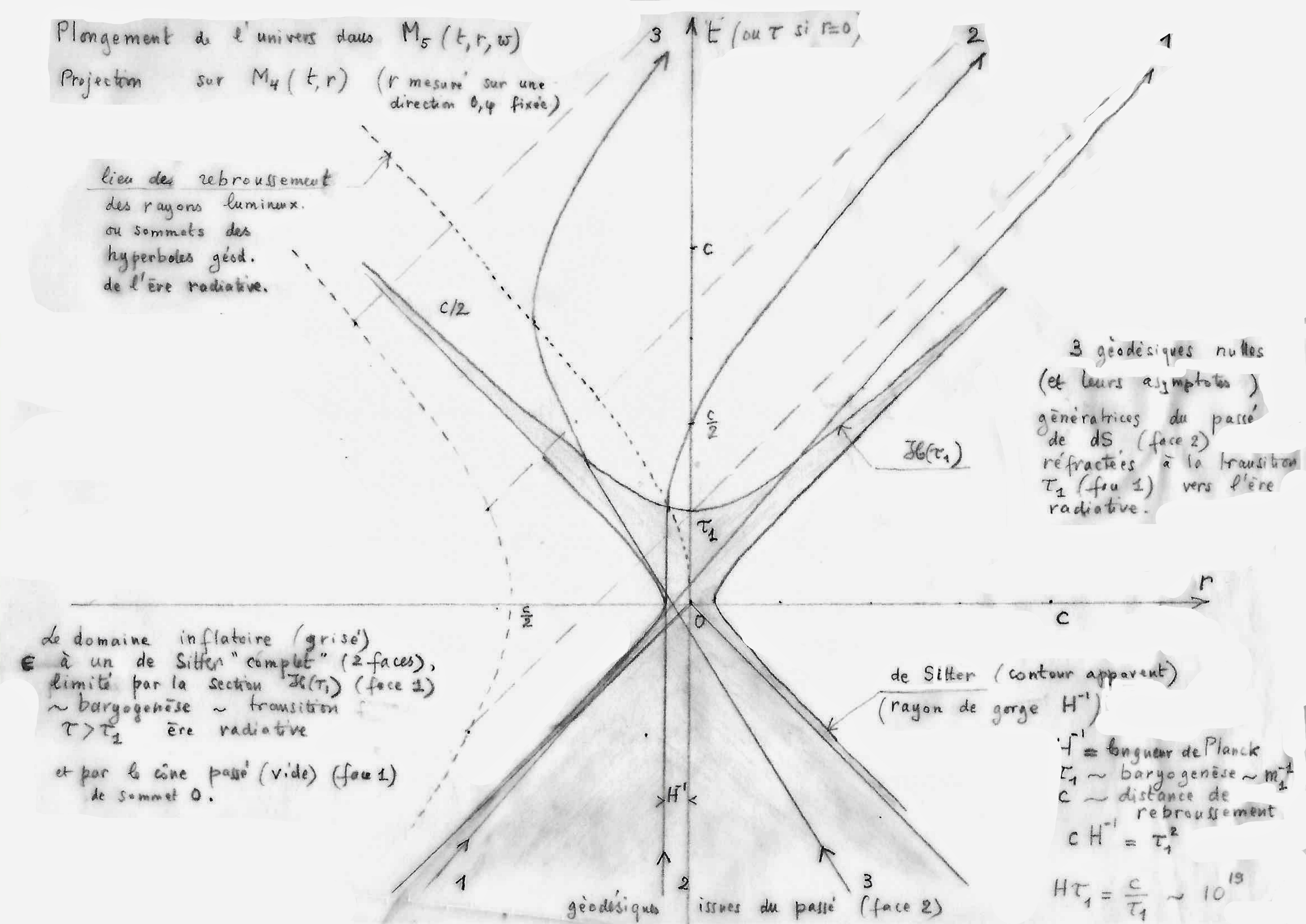
Michel Gaudin was an original and prolific personality. He was principally guided by a quest for elegance, and little cared for recognition. (In 2019 he was, however, awarded the prestigious Dannie Heinemann Prize for Mathematical Physics.) He published in French and paid particular attention to the style of his writing. He was a passionate of classical culture, in particular of literature and philosophy. We see him again reading the "Mémoires d'outre-tombe", looking on a map for Chateaubriand's stops. In conversation, he liked to recall verses from Racine or La Fontaine. He was also a man of great faith. He considered himself a craftsman, and was convinced that that the hand is the support of thought. This translated into scientific texts written in a quite literary style and drafted in beautiful handwriting, sometimes accompanied by drawings on graph paper. To convey the idea of his meticulousness, we enclose a drawing of geodesic trajectories done by Gaudin shortly before his death.
A master and a friend has left us.