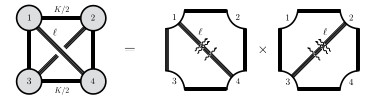
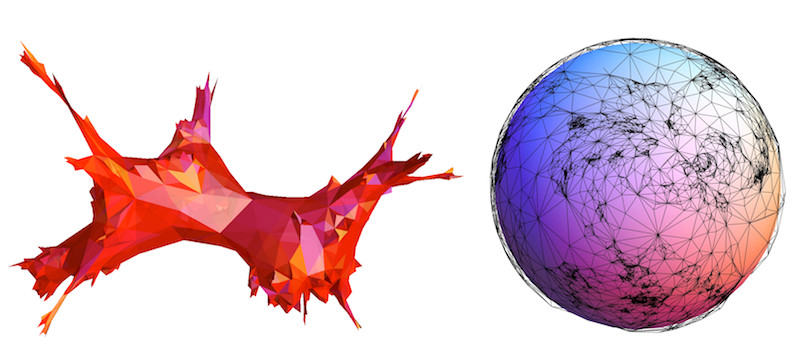
La physique mathématique couvre un large éventail de sujets en physique théorique, allant des mathématiques presque pures à certaines études qui peuvent impliquer des expériences. Malgré cette diversité, beaucoup de ces sujets sont liés par le fait qu’ils utilisent des outils mathématiques et théoriques communs (théorie quantique des champs, systèmes intégrables, théorie conforme des champs, théorie des cordes, matrices aléatoires, combinatoire, probabilités et processus stochastiques), et que la rigueur et la cohérence mathématiques, ainsi que l’obtention de résultats précis et souvent explicites, sont très importantes.
Beaucoup de ces outils ont été développés au fil des années à l’IPhT. Sur plusieurs sujets, les contacts et les collaborations entre l’IPhT et les mathématiciens se développent rapidement. Cela ne reflète pas un mouvement vers plus d’abstraction, mais plutôt un véritable intérêt des mathématiciens pour les problèmes de physique, et des physiciens pour les idées et les nouveaux outils mathématiques développés par les mathématiciens. La physique mathématique à l’IPhT a également des contacts étroits avec la physique statistique et la physique des hautes énergies. Par exemple, des progrès importants sont réalisés sur le problème de l’évaluation des amplitudes de diffusion, qui trouve aujourd’hui sa place dans la phénoménologie du LHC, dans la cosmologie et dans la physique statistique.